The Universe in the Heel of a Sock

Little-known fact about socks: The mathematics that determines the heel curvature of hosiery has its origins in Einstein’s theory of relativity, which describes curved space-time. So the math and science you learned in high school actually do have real-life applications after all.

Physicist Sabetta Matsumoto, a visiting scholar at UC Santa Barbara’s Kavli Institute for Theoretical Physics (KITP), unpacked the math of curvature in easily understandable terms in her presentation “Purls of Wisdom.” Her talk, part of the ongoing science series Café KITP, addressed the geometry and topology used in sewing garments and knitting clothes.

“The human form — particularly the female body — has innate curvature,” said Matsumoto, currently a postdoctoral scholar in the Applied Mathematics Group at Harvard University. “Fabric is two-dimensional, yet seamstresses, couturiers and dressmakers all know how to make it fit a three-dimensional body by adding darts and pleats, which physicists like to call topological defects. Darts in clothing are an example of positive curvature, where area is removed.”

Matsumoto comes by her penchant for fiber arts genetically. Her mother is a fiber artist who taught her to sew and knit. Matsumoto used her own designer wedding dress as part of her slideshow, comparing its ruffles to human intestines.

“You might think that these two things have absolutely nothing in common, but surprisingly, it is actually the same physical mechanism that gives both their shape —negative curvature,” Matsumoto said. In negative curvature, the surface curves away in two different directions in the same way a saddle or a Pringles potato chip does.

Matsumoto’s ruffled wedding dress was created by stretching tulle — adding extra area — and stitching it to stiff boning. When the tension on the fabric was relaxed, a ruffled structure appeared. “It turns out that the same thing is going on in this fabric treatment and in your intestines,” Matsumoto explained. “A small membrane that connects the intestines to the spine created tension when your intestines grew and ruffled them.”

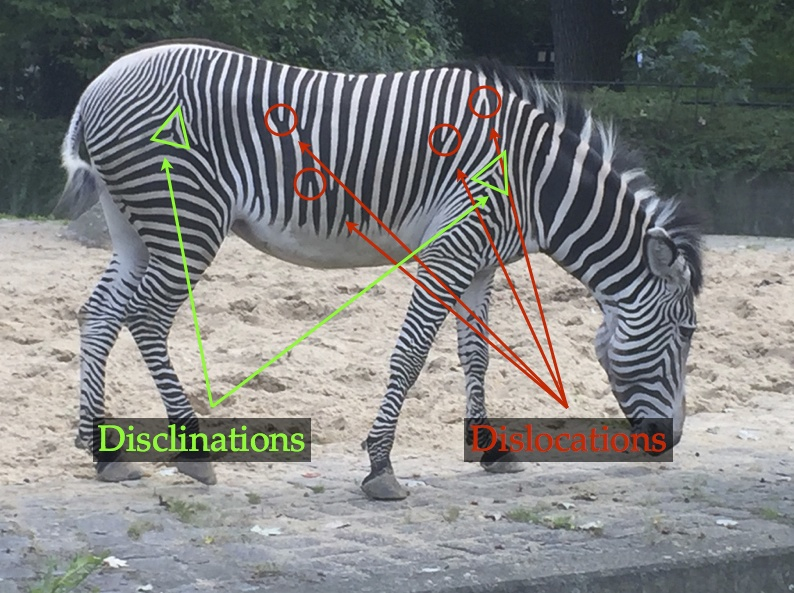
Matsumoto also used knitting to discuss two categories of topological defects: dislocation and disclination. “Dislocations are where we add in new columns of material — for instance, on the bustline of a sweater," she said. “But you also see dislocations when you knit in new rows of material —this is called adding in short rows — and this is exactly what’s going on in the heel of a sock.”

Both dislocation and disclination can also be seen in the stripe pattern of a zebra. The dislocations are areas where the girth of the animal changes and a new stripe is added. The disclinations are areas where curvature changes to accommodate the animal’s limbs.

At the core of Matsumoto’s presentation was her handmade fabric rendition of the hyperbolic plane geometry known as Klein’s Quartic Curve. Her creation consists of 24 heptagons (seven-sided flat pieces) coordinated in groups of three. “I took all this space and I wrapped it in on itself to come up with a compact object containing three holes,” Matsumoto said.

Matsumoto also walked the audience through the math of Euler’s formula in order to demonstrate the deep relationship between the geometry (the counting of edges) and the topology of the object (the number of holes).

“You may have remembered your geometry classroom as something dry, but I hope I’ve convinced you that if you keep your eyes open, you can find interesting geometry all around you in your daily life,” Matsumoto concluded.